My Favorite Math Videos: Part 2
- Catherine

- Jul 12, 2020
- 6 min read
Updated: Dec 16, 2020
Welcome back to GLeaM!
As a bit of an update, I'm currently participating in an online summer math program called Ross, and as a result, I'm going on an every-other-week schedule for GLeaM articles. Stay tuned though—because I have a lot of exciting article ideas because of Ross: everything from how internet cryptography works to how to problem-solve like a pro. I've been reading some math books in an effort to bring you some new exciting content, so we'll see where it takes us!
Ross has been such a cool experience so far, and I encourage you to seek out your own math-themed opportunities even if you're stuck at home; in fact, I even have an article all about ideas for math activities you can pursue during quarantine: check it out here!
As for today's article, I know that many of you enjoyed my last read-and-watch article, all about my favorite math videos, so I thought I'd deliver a part 2!
Many of these videos were mentioned in my honorable mentions on that first article, so if you're really invested, you might have already seen them, but either way, I thought they deserved a larger spotlight! And of course, there are also some videos I've recently discovered on this list as well!
So without further ado, in no particular order, here's 8 more of my favorite math videos!
1) Sneaky Topology - 3Blue1Brown
I'll start with a video that actually ended up inspiring me to complete one of my favorite mathematical projects to date.
Say you and a friend steal a necklace with a certain number of jewels on it: rubies, diamonds, sapphires, emeralds, etc. Say that there is an even number of each type of jewel on the necklace and there are n types of jewels. Is there always a way to split the necklace with only n cuts such that each of you can take some of the cut segments and have exactly half of every type of jewel?
Now, let me ask a seemingly unrelated question. Imagine you have a hollow sphere in 3D space and you decide to squash it down so it's flat, aka so it fits in 2D space. Will there always be a pair of points that land on top of each other no matter how you squash the sphere?
This second statement comes from a field called topology: essentially, the study of surfaces and how they function regardless of their exact shape. For most purposes, we'd consider a sphere that is hollow to behave similarly to a cube that is hollow or a cone is hollow—they both have one hidden hole. Similarly, a donut is the same as a coffee cup, they both have exactly one hole—and you can morph one into the other without cutting or gluing the surface!
In topology, we care about what happens when we manipulate those surfaces. Do they stretch, do they intersect themselves, do they change? What properties does a surface maintain no matter what you do to it, as long as you don't cut it or glue it?
Sure, the two questions we stated are fascinating in their own right, but what's even more fascinating is they're extremely intertwined. In fact, you can solve one with the other.
The video itself is by the award-winning animator and math lover, 3Blue1Brown, aka Grant Sanderson. He actually programs all of his own animations and puts so much work into his videos to explain everything clearly, so they never disappoint!
It is a very complicated topic, so don't worry if you don't understand it all on the first—or even the tenth—watch. Math always takes time.
This video inspired me to spend a week exploring these ideas at Georgia's Governor's Honors Program last summer, and it was so much fun!
That was quite a long introduction, so here you go!
2) The Brick Double Domino Effect - standupmaths
This video is from Matt Parker, who wrote the book Things to Make and Do in the Fourth Dimension. He considers himself a standup mathematician: a comedian and a mathematician.
This specific video is all about what happens when you set up a chain of bricks like dominos and knock them over—in a very particular way. It turns out, dominos can fall backwards too! I won't give too much away, so go ahead and watch it!
3) How to Choose a Toilet - Numberphile
Featuring the amazing female mathematician Dr. Ria Symonds from the University of Nottingham, this Numberphile video covers the famous Secretary Problem.
Say you're interviewing 100 people for one secretary position. You have to either accept or reject each person immediately after their interview. What strategy should you have to ensure the highest likelihood of accepting the best person out of the 100 for your position?
It's a really interesting problem in its own right, and this video frames it in another context: Given 100 porta potties at a music festival, what strategy should you have to ensure the highest likelihood of choosing the best one to use?
I'm hoping to write an article on this problem, so stay tuned!
4) Who cares about topology? - 3Blue1Brown
This video is yet another topology video by 3Blue1Brown, but it's very different and just as fascinating as the first one!
There's an unsolved conjecture in math right now that given any closed loop, like so, you can always inscribe at least one square in the loop:
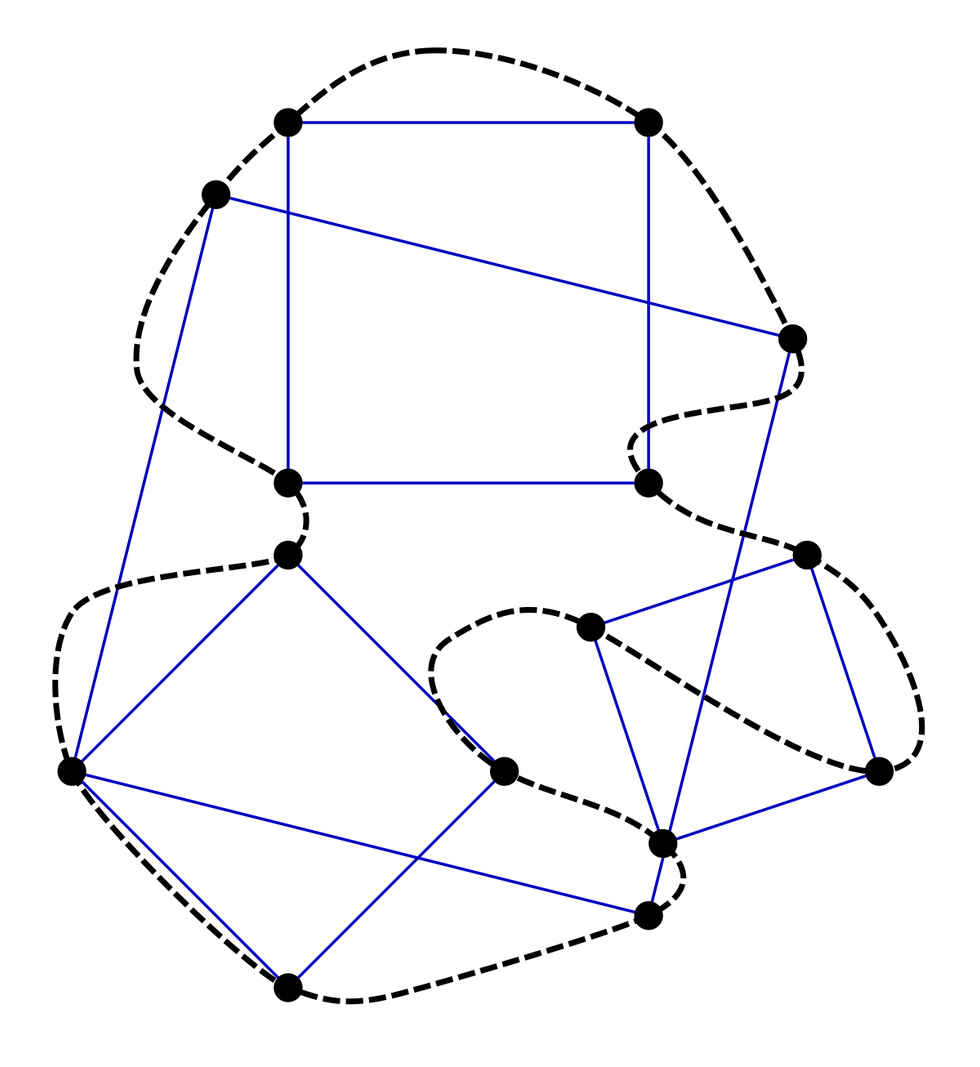
However, though we can't solve the problem for squares, we can solve it for a more general case: There does happen to be an inscribed rectangle in any closed loop, and who could guess that the path we take to get there somehow involves 3D surfaces and Möbius strips??
3Blue1Brown actually claims this is his favorite piece of math ever, so check it out!
5) The Stable Marriage Problem - Numberphile
Imagine you were a matchmaker in charge of choosing marriages between four princes and princesses, and you knew each of the princes' preferences between the princesses and each of the princesses' preferences between the princes.
You can choose any combinations of marriages you like, as long as there is not a prince and princess who both prefer each other to their matches—because then they'd run off together!
(For example, if Alice prefers Bob to Charlie and Bob prefers Alice to Donna, you can't match Alice with Charlie and Bob with Donna. This is what makes the marriages stable.)
It turns out that this problem is actually extremely applicable (in different forms, of course) across computer science, economics and even college admissions.
Hear it from Dr. Emily Riehl of Johns Hopkins University here:
6) Infinity is bigger than you think - Numberphile
I'm always willing to talk about infinity. In fact, I already have two articles about infinity and the fact that some infinities are actually larger than others! Check out "What Would an Infinite Hotel Look Like?" here, and "Are Some Infinities Bigger than Others?" here.
As for this particular video, it's an explanation of why there may be more real numbers than rational numbers from James Grime, who actually runs his own successful math YouTube channel: singingbanana.
It's such an articulate, passionate, clear explanation, so all around just a brilliant video!
7) Doodling in Math: Spirals, Fibonacci, and Being a Plant - Vi Hart
Vi Hart's videos are so creative and insightful. She's actually a self-proclaimed mathemusician because many of her videos are musically themed.
I really like her series on the Fibonacci sequence because she does a great job delving into golden spirals and how Fibonacci numbers show up in nature, all while keeping the videos super entertaining. All of these videos have over a million views (and the first close to five million) so they've gone borderline viral as well, which is always great to see.
It's a three-part series, so I'll leave the first video here and link the other two below:
If you'd like to see even more about Fibonacci and the Golden Ratio, check out of one of my early quarantine articles: "Good as Gold: The Fibonacci Sequence, the Golden Ratio, and More!"
8) Stand-up comedy about Equations that Correspond to Vortex Motions (aka "smoke rings") - standupmaths
Whew, what a mouthful of a title! This video features Matt Parker, the standup mathematician from earlier in this article, presenting about vortex motions at the Festival of the Spoken Nerd.
But really, it's much more than that. You get to witness a terrible feud between mathematicians and physicists and see giant donut-shaped tornados being spawned into a crowded audience! You even get to see a mathematician use a garden bin as a gun to knock cups off people's heads! This video is quite chaotic, but stick with it because they'll move from the equations to some really incredible demonstrations.
Yes, I've recommended one of Matt Parker's standup comedy routines in my last article of this form, but hey, they're pretty fun to watch! I'd have to say my favorite is still the one in the last article, so check out Matt Parker's comedy routine about spreadsheets at the below link:
CONCLUSION
Of course, there are more amazing math videos out there, so I encourage you to go exploring yourself! The resources section of my site (you'll have to become a member to see it!) has a lot of great suggestions for channels, and you can always check out "My Favorite Math Videos: Part 1" for eight more descriptions—and even honorable mentions!
It's been super fun compiling these for you guys today, and I'll see you in two weeks! In the meantime, feel free to contact me—in the comments, through the contact form, or through the forum—if you have any questions or need any math-related advice. I encourage you to use the community to talk to one another too! Have a great week everybody!




Comments